CoordinateSystem#

Qualified name: manim.mobject.graphing.coordinate\_systems.CoordinateSystem

class CoordinateSystem(x_range=None, y_range=None, x_length=None, y_length=None, dimension=2)[source]#

Bases: object

Abstract base class for Axes and NumberPlane.

Examples

Example: CoordSysExample ¶

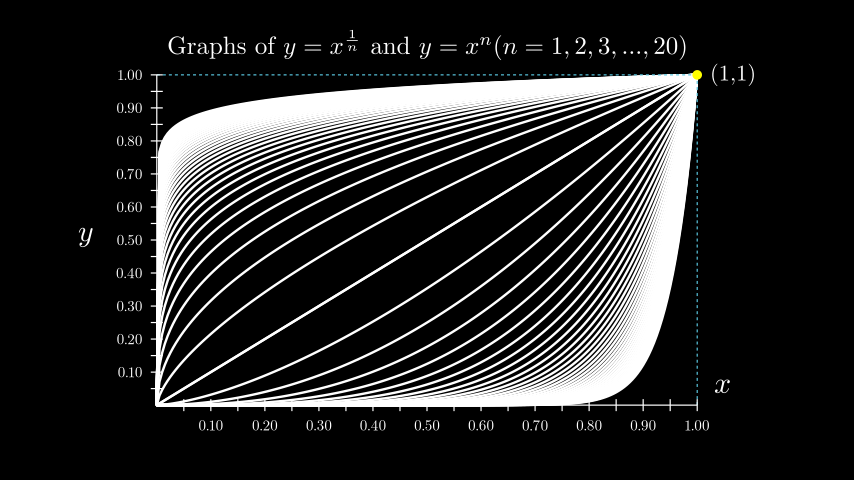
from manim import *

class CoordSysExample(Scene):
    def construct(self):
        # the location of the ticks depends on the x_range and y_range.
        grid = Axes(
            x_range=[0, 1, 0.05],  # step size determines num_decimal_places.
            y_range=[0, 1, 0.05],
            x_length=9,
            y_length=5.5,
            axis_config={
                "numbers_to_include": np.arange(0, 1 + 0.1, 0.1),
                "font_size": 24,
            },
            tips=False,
        )

        # Labels for the x-axis and y-axis.
        y_label = grid.get_y_axis_label("y", edge=LEFT, direction=LEFT, buff=0.4)
        x_label = grid.get_x_axis_label("x")
        grid_labels = VGroup(x_label, y_label)

        graphs = VGroup()
        for n in np.arange(1, 20 + 0.5, 0.5):
            graphs += grid.plot(lambda x: x ** n, color=WHITE)
            graphs += grid.plot(
                lambda x: x ** (1 / n), color=WHITE, use_smoothing=False
            )

        # Extra lines and labels for point (1,1)
        graphs += grid.get_horizontal_line(grid.c2p(1, 1, 0), color=BLUE)
        graphs += grid.get_vertical_line(grid.c2p(1, 1, 0), color=BLUE)
        graphs += Dot(point=grid.c2p(1, 1, 0), color=YELLOW)
        graphs += Tex("(1,1)").scale(0.75).next_to(grid.c2p(1, 1, 0))
        title = Title(
            # spaces between braces to prevent SyntaxError
            r"Graphs of $y=x^{ {1}\over{n} }$ and $y=x^n (n=1,2,3,...,20)$",
            include_underline=False,
            font_size=40,
        )

        self.add(title, graphs, grid, grid_labels)

Methods

`add_coordinates`	Adds labels to the axes.
`angle_of_tangent`	Returns the angle to the x-axis of the tangent to the plotted curve at a particular x-value.
`c2p`	Abbreviation for `coords_to_point()`
`coords_to_point`
`get_T_label`	Creates a labelled triangle marker with a vertical line from the x-axis to a curve at a given x-value.
`get_area`	Returns a `Polygon` representing the area under the graph passed.
`get_axes`
`get_axis`
`get_axis_labels`
`get_graph_label`	Creates a properly positioned label for the passed graph, with an optional dot.
`get_horizontal_line`	A horizontal line from the y-axis to a given point in the scene.
`get_line_from_axis_to_point`	Returns a straight line from a given axis to a point in the scene.
`get_lines_to_point`	Generate both horizontal and vertical lines from the axis to a point.
`get_origin`	Gets the origin of `Axes`.
`get_riemann_rectangles`	Generates a `VGroup` of the Riemann Rectangles for a given curve.
`get_secant_slope_group`	Creates two lines representing dx and df, the labels for dx and df, and
`get_vertical_line`	A vertical line from the x-axis to a given point in the scene.
`get_vertical_lines_to_graph`	Obtains multiple lines from the x-axis to the curve.
`get_x_axis`
`get_x_axis_label`	Generate an x-axis label.
`get_x_unit_size`
`get_y_axis`
`get_y_axis_label`	Generate a y-axis label.
`get_y_unit_size`
`get_z_axis`
`i2gc`	Alias for `input_to_graph_coords()`.
`i2gp`	Alias for `input_to_graph_point()`.
`input_to_graph_coords`	Returns a tuple of the axis relative coordinates of the point on the graph based on the x-value given.
`input_to_graph_point`	Returns the coordinates of the point on a `graph` corresponding to an `x` value.
`p2c`	Abbreviation for `point_to_coords()`
`plot`	Generates a curve based on a function.
`plot_antiderivative_graph`	Plots an antiderivative graph.
`plot_derivative_graph`	Returns the curve of the derivative of the passed graph.
`plot_implicit_curve`	Creates the curves of an implicit function.
`plot_parametric_curve`	A parametric curve.
`plot_polar_graph`	A polar graph.
`plot_surface`	Generates a surface based on a function.
`point_to_coords`
`point_to_polar`	Gets polar coordinates from a point.
`polar_to_point`	Gets a point from polar coordinates.
`pr2pt`	Abbreviation for `polar_to_point()`
`pt2pr`	Abbreviation for `point_to_polar()`
`slope_of_tangent`	Returns the slope of the tangent to the plotted curve at a particular x-value.

add_coordinates(*axes_numbers, **kwargs)[source]#

Adds labels to the axes. Use Axes.coordinate_labels to access the coordinates after creation.

Parameters: axes_numbers (Iterable[float] | None | dict[float, str | float | Mobject]) – The numbers to be added to the axes. Use None to represent an axis with default labels.

Examples

ax = ThreeDAxes()
x_labels = range(-4, 5)
z_labels = range(-4, 4, 2)
ax.add_coordinates(x_labels, None, z_labels)  # default y labels, custom x & z labels
ax.add_coordinates(x_labels)  # only x labels

You can also specifically control the position and value of the labels using a dict.

ax = Axes(x_range=[0, 7])
x_pos = [x for x in range(1, 8)]

# strings are automatically converted into a Tex mobject.
x_vals = ["Monday", "Tuesday", "Wednesday", "Thursday", "Friday", "Saturday", "Sunday"]
x_dict = dict(zip(x_pos, x_vals))
ax.add_coordinates(x_dict)

angle_of_tangent(x, graph, dx=1e-08)[source]#

Returns the angle to the x-axis of the tangent to the plotted curve at a particular x-value.

Parameters

x (float) – The x-value at which the tangent must touch the curve.
graph (ParametricFunction) – The ParametricFunction for which to calculate the tangent.
dx (float) – The change in x used to determine the angle of the tangent to the curve.

Returns

The angle of the tangent to the curve.

Return type

float

Examples

ax = Axes()
curve = ax.plot(lambda x: x**2)
ax.angle_of_tangent(x=3, graph=curve)
# 1.4056476493802699

c2p(*coords)[source]#: Abbreviation for coords_to_point()

get_T_label(x_val, graph, label=None, label_color=None, triangle_size=0.25, triangle_color='#FFFFFF', line_func=<class 'manim.mobject.geometry.line.Line'>, line_color='#FFFF00')[source]#

Creates a labelled triangle marker with a vertical line from the x-axis to a curve at a given x-value.

Parameters

x_val (float) – The position along the curve at which the label, line and triangle will be constructed.
graph (ParametricFunction) – The ParametricFunction for which to construct the label.
label (float | str | Mobject | None) – The label of the vertical line and triangle.
label_color (Color | None) – The color of the label.
triangle_size (float) – The size of the triangle.
triangle_color (Color | None) – The color of the triangle.
line_func (Line) – The function used to construct the vertical line.
line_color (Color) – The color of the vertical line.

Returns

A VGroup of the label, triangle and vertical line mobjects.

Return type

VGroup

Examples

Example: TLabelExample ¶

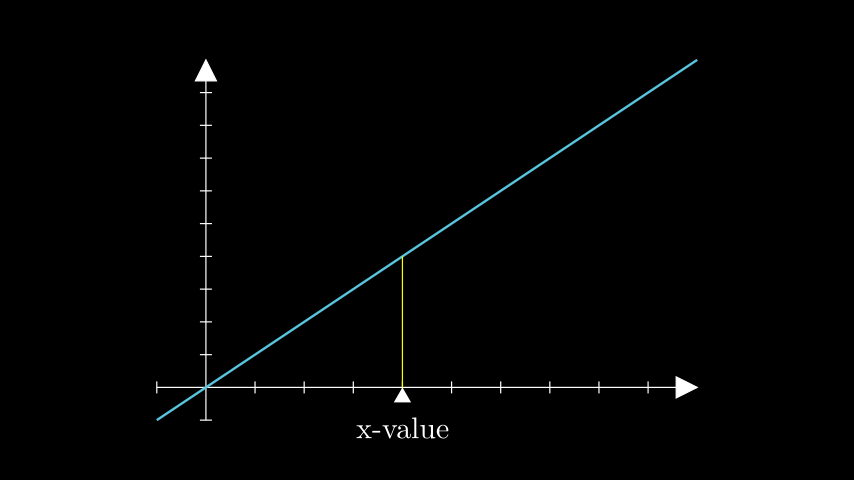
from manim import *

class TLabelExample(Scene):
    def construct(self):
        # defines the axes and linear function
        axes = Axes(x_range=[-1, 10], y_range=[-1, 10], x_length=9, y_length=6)
        func = axes.plot(lambda x: x, color=BLUE)
        # creates the T_label
        t_label = axes.get_T_label(x_val=4, graph=func, label=Tex("x-value"))
        self.add(axes, func, t_label)

get_area(graph, x_range=None, color=['#58C4DD', '#83C167'], opacity=0.3, bounded_graph=None, **kwargs)[source]#

Returns a Polygon representing the area under the graph passed.

Parameters

graph (ParametricFunction) – The graph/curve for which the area needs to be gotten.
x_range (tuple[float, float] | None) – The range of the minimum and maximum x-values of the area. x_range = [x_min, x_max].
color (Color | Iterable[Color]) – The color of the area. Creates a gradient if a list of colors is provided.
opacity (float) – The opacity of the area.
bounded_graph (ParametricFunction) – If a secondary graph is specified, encloses the area between the two curves.
kwargs – Additional parameters passed to Polygon.

Returns

The Polygon representing the area.

Return type

Polygon

Raises

ValueError – When x_ranges do not match (either area x_range, graph’s x_range or bounded_graph’s x_range).

Examples

Example: GetAreaExample ¶

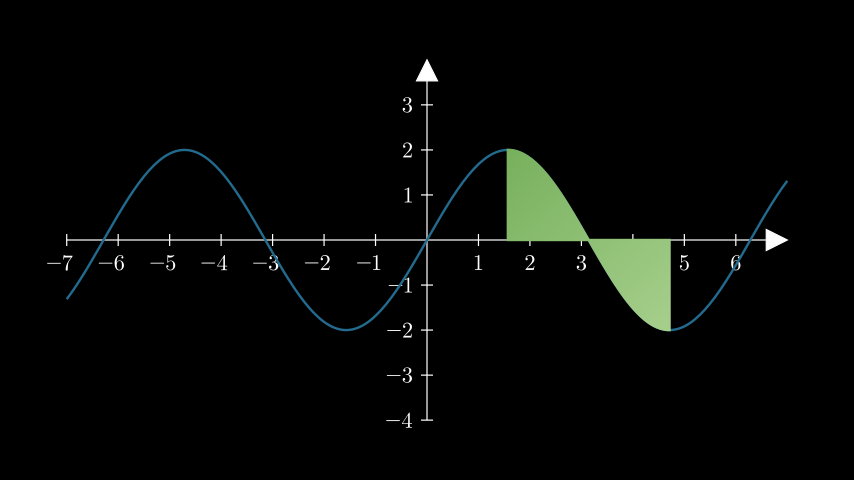
from manim import *

class GetAreaExample(Scene):
    def construct(self):
        ax = Axes().add_coordinates()
        curve = ax.plot(lambda x: 2 * np.sin(x), color=DARK_BLUE)
        area = ax.get_area(
            curve,
            x_range=(PI / 2, 3 * PI / 2),
            color=(GREEN_B, GREEN_D),
            opacity=1,
        )

        self.add(ax, curve, area)

get_graph_label(graph, label='f(x)', x_val=None, direction=array([1., 0., 0.]), buff=0.25, color=None, dot=False, dot_config=None)[source]#

Creates a properly positioned label for the passed graph, with an optional dot.

Parameters

graph (ParametricFunction) – The curve.
label (float | str | Mobject) – The label for the function’s curve. Defaults to MathTex for str and float inputs.
x_val (float | None) – The x_value along the curve that positions the label.
direction (Sequence[float]) – The cartesian position, relative to the curve that the label will be at –> LEFT, RIGHT.
buff (float) – The distance between the curve and the label.
color (Color | None) – The color of the label. Defaults to the color of the curve.
dot (bool) – Whether to add a dot at the point on the graph.
dot_config (dict | None) – Additional parameters to be passed to Dot.

Returns

The positioned label and Dot, if applicable.

Return type

Mobject

Examples

Example: GetGraphLabelExample ¶

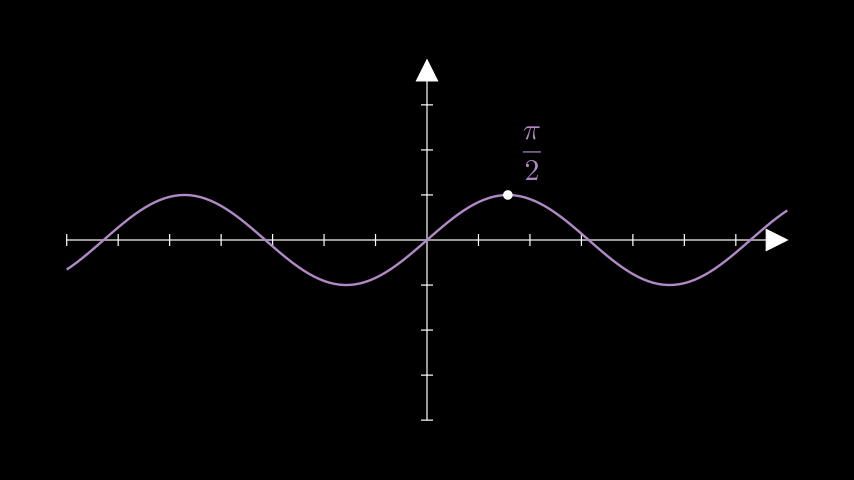
from manim import *

class GetGraphLabelExample(Scene):
    def construct(self):
        ax = Axes()
        sin = ax.plot(lambda x: np.sin(x), color=PURPLE_B)
        label = ax.get_graph_label(
            graph=sin,
            label= MathTex(r"\frac{\pi}{2}"),
            x_val=PI / 2,
            dot=True,
            direction=UR,
        )

        self.add(ax, sin, label)

get_horizontal_line(point, **kwargs)[source]#

A horizontal line from the y-axis to a given point in the scene.

Parameters

point (Sequence[float]) – The point to which the horizontal line will be drawn.
kwargs – Additional parameters to be passed to get_line_from_axis_to_point.

Returns

A horizontal line from the y-axis to the point.

Return type

Line

Examples

Example: GetHorizontalLineExample ¶

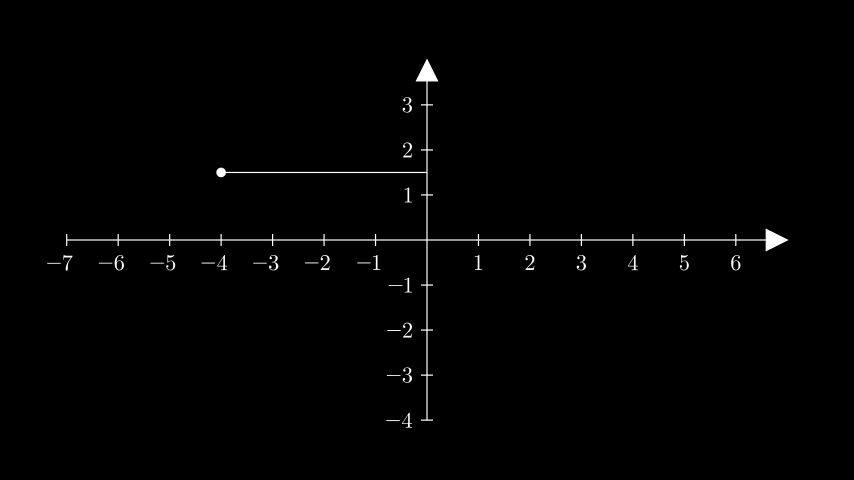
../_images/GetHorizontalLineExample-1.png

from manim import *

class GetHorizontalLineExample(Scene):
    def construct(self):
        ax = Axes().add_coordinates()
        point = ax.c2p(-4, 1.5)

        dot = Dot(point)
        line = ax.get_horizontal_line(point, line_func=Line)

        self.add(ax, line, dot)

get_line_from_axis_to_point(index, point, line_func=<class 'manim.mobject.geometry.line.DashedLine'>, line_config=None, color=None, stroke_width=2)[source]#

Returns a straight line from a given axis to a point in the scene.

Parameters

index (int) – Specifies the axis from which to draw the line. 0 = x_axis, 1 = y_axis
point (Sequence[float]) – The point to which the line will be drawn.
line_func (Line) – The function of the Line mobject used to construct the line.
line_config (dict | None) – Optional arguments to passed to line_func.
color (Color | None) – The color of the line.
stroke_width (float) – The stroke width of the line.

Returns

The line from an axis to a point.

Return type

Line

Examples

Example: GetLinesToPointExample ¶

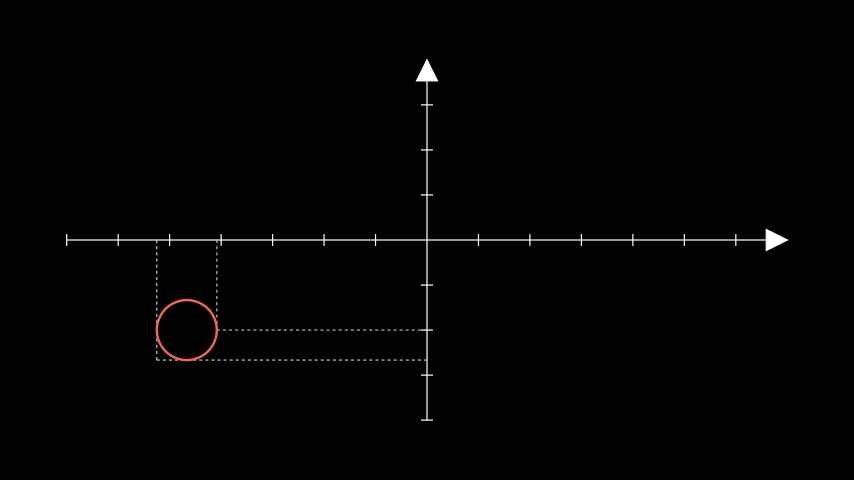
from manim import *

class GetLinesToPointExample(Scene):
    def construct(self):
        ax = Axes()
        circ = Circle(radius=0.5).move_to([-4, -1.5, 0])

        lines_1 = ax.get_lines_to_point(circ.get_right(), color=GREEN_B)
        lines_2 = ax.get_lines_to_point(circ.get_corner(DL), color=BLUE_B)
        self.add(ax, lines_1, lines_2, circ)

get_origin()[source]#

Gets the origin of Axes.

Returns: The center point.
Return type: np.ndarray

get_riemann_rectangles(graph, x_range=None, dx=0.1, input_sample_type='left', stroke_width=1, stroke_color='#000000', fill_opacity=1, color=array(['#58C4DD', '#83C167'], dtype='<U7'), show_signed_area=True, bounded_graph=None, blend=False, width_scale_factor=1.001)[source]#

Generates a VGroup of the Riemann Rectangles for a given curve.

Parameters

graph (ParametricFunction) – The graph whose area will be approximated by Riemann rectangles.
x_range (Sequence[float] | None) – The minimum and maximum x-values of the rectangles. x_range = [x_min, x_max].
dx (float | None) – The change in x-value that separates each rectangle.
input_sample_type (str) – Can be any of "left", "right" or "center". Refers to where the sample point for the height of each Riemann Rectangle will be inside the segments of the partition.
stroke_width (float) – The stroke_width of the border of the rectangles.
stroke_color (Color) – The color of the border of the rectangle.
fill_opacity (float) – The opacity of the rectangles.
color (Iterable[Color] | Color) – The colors of the rectangles. Creates a balanced gradient if multiple colors are passed.
show_signed_area (bool) – Indicates negative area when the curve dips below the x-axis by inverting its color.
blend (bool) – Sets the stroke_color to fill_color, blending the rectangles without clear separation.
bounded_graph (ParametricFunction) – If a secondary graph is specified, encloses the area between the two curves.
width_scale_factor (float) – The factor by which the width of the rectangles is scaled.

Returns

A VGroup containing the Riemann Rectangles.

Return type

VGroup

Examples

Example: GetRiemannRectanglesExample ¶

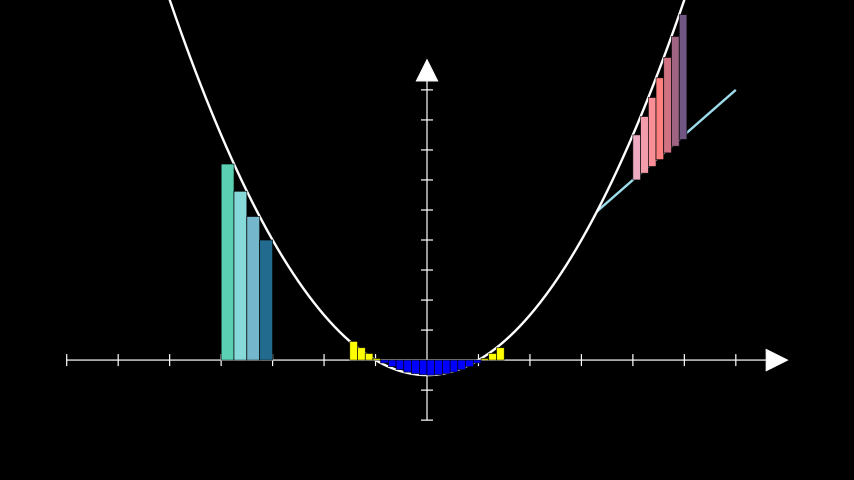
../_images/GetRiemannRectanglesExample-1.png

from manim import *

class GetRiemannRectanglesExample(Scene):
    def construct(self):
        ax = Axes(y_range=[-2, 10])
        quadratic = ax.plot(lambda x: 0.5 * x ** 2 - 0.5)

        # the rectangles are constructed from their top right corner.
        # passing an iterable to `color` produces a gradient
        rects_right = ax.get_riemann_rectangles(
            quadratic,
            x_range=[-4, -3],
            dx=0.25,
            color=(TEAL, BLUE_B, DARK_BLUE),
            input_sample_type="right",
        )

        # the colour of rectangles below the x-axis is inverted
        # due to show_signed_area
        rects_left = ax.get_riemann_rectangles(
            quadratic, x_range=[-1.5, 1.5], dx=0.15, color=YELLOW
        )

        bounding_line = ax.plot(
            lambda x: 1.5 * x, color=BLUE_B, x_range=[3.3, 6]
        )
        bounded_rects = ax.get_riemann_rectangles(
            bounding_line,
            bounded_graph=quadratic,
            dx=0.15,
            x_range=[4, 5],
            show_signed_area=False,
            color=(MAROON_A, RED_B, PURPLE_D),
        )

        self.add(
            ax, bounding_line, quadratic, rects_right, rects_left, bounded_rects
        )

get_secant_slope_group(x, graph, dx=None, dx_line_color='#FFFF00', dy_line_color=None, dx_label=None, dy_label=None, include_secant_line=True, secant_line_color='#83C167', secant_line_length=10)[source]#

Creates two lines representing dx and df, the labels for dx and df, and: the secant to the curve at a particular x-value.

Parameters

x (float) – The x-value at which the secant intersects the graph for the first time.
graph (ParametricFunction) – The curve for which the secant will be found.
dx (float | None) – The change in x after which the secant exits.
dx_line_color (Color) – The color of the line that indicates the change in x.
dy_line_color (Color | None) – The color of the line that indicates the change in y. Defaults to the color of graph.
dx_label (float | str | None) – The label for the dx line. Defaults to MathTex for str and float inputs.
dy_label (float | str | None) – The label for the dy line. Defaults to MathTex for str and float inputs.
include_secant_line (bool) – Whether to include the secant line in the graph, or just the df/dx lines and labels.
secant_line_color (Color) – The color of the secant line.
secant_line_length (float) – The length of the secant line.

Returns

A group containing the elements: dx_line, df_line, and if applicable also dx_label, df_label, secant_line.

Return type

VGroup

Examples

Example: GetSecantSlopeGroupExample ¶

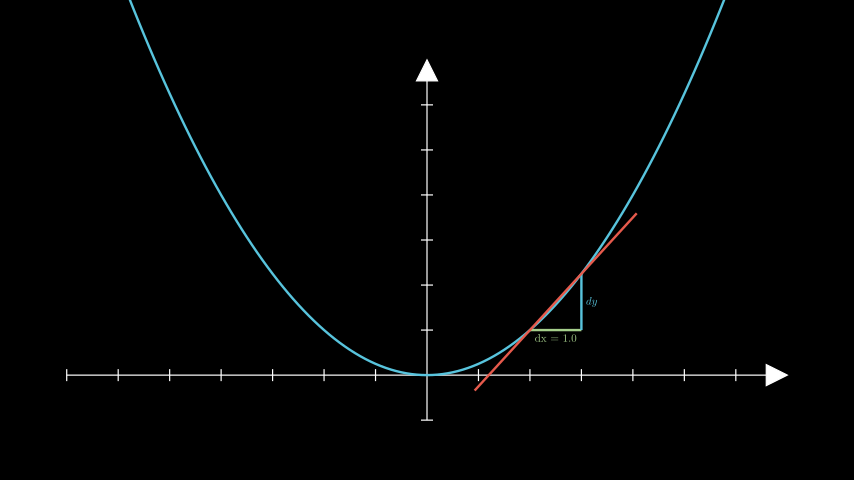
../_images/GetSecantSlopeGroupExample-1.png

from manim import *

class GetSecantSlopeGroupExample(Scene):
    def construct(self):
        ax = Axes(y_range=[-1, 7])
        graph = ax.plot(lambda x: 1 / 4 * x ** 2, color=BLUE)
        slopes = ax.get_secant_slope_group(
            x=2.0,
            graph=graph,
            dx=1.0,
            dx_label=Tex("dx = 1.0"),
            dy_label="dy",
            dx_line_color=GREEN_B,
            secant_line_length=4,
            secant_line_color=RED_D,
        )

        self.add(ax, graph, slopes)

get_vertical_line(point, **kwargs)[source]#

A vertical line from the x-axis to a given point in the scene.

Parameters

point (Sequence[float]) – The point to which the vertical line will be drawn.
kwargs – Additional parameters to be passed to get_line_from_axis_to_point.

Returns

A vertical line from the x-axis to the point.

Return type

Line

Examples

Example: GetVerticalLineExample ¶

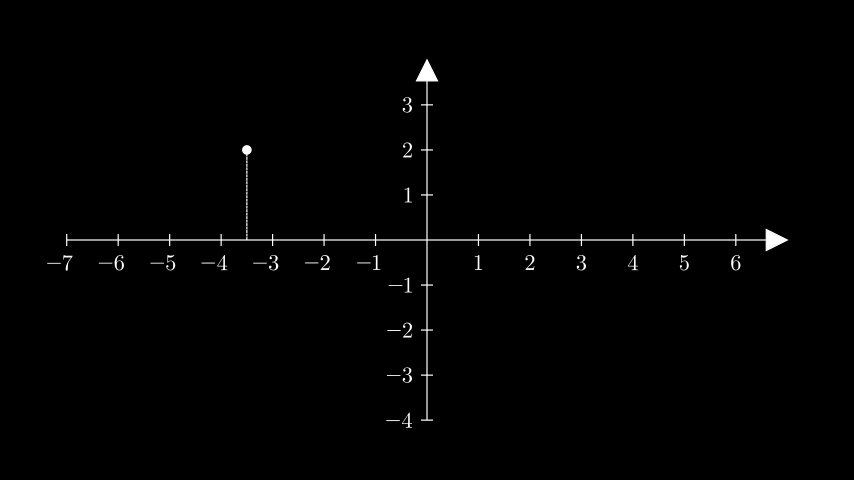
from manim import *

class GetVerticalLineExample(Scene):
    def construct(self):
        ax = Axes().add_coordinates()
        point = ax.coords_to_point(-3.5, 2)

        dot = Dot(point)
        line = ax.get_vertical_line(point, line_config={"dashed_ratio": 0.85})

        self.add(ax, line, dot)

get_vertical_lines_to_graph(graph, x_range=None, num_lines=20, **kwargs)[source]#

Obtains multiple lines from the x-axis to the curve.

Parameters

graph (ParametricFunction) – The graph along which the lines are placed.
x_range (Sequence[float] | None) – A list containing the lower and and upper bounds of the lines: x_range = [x_min, x_max].
num_lines (int) – The number of evenly spaced lines.
kwargs – Additional arguments to be passed to get_vertical_line().

Returns

The VGroup of the evenly spaced lines.

Return type

VGroup

Examples

Example: GetVerticalLinesToGraph ¶

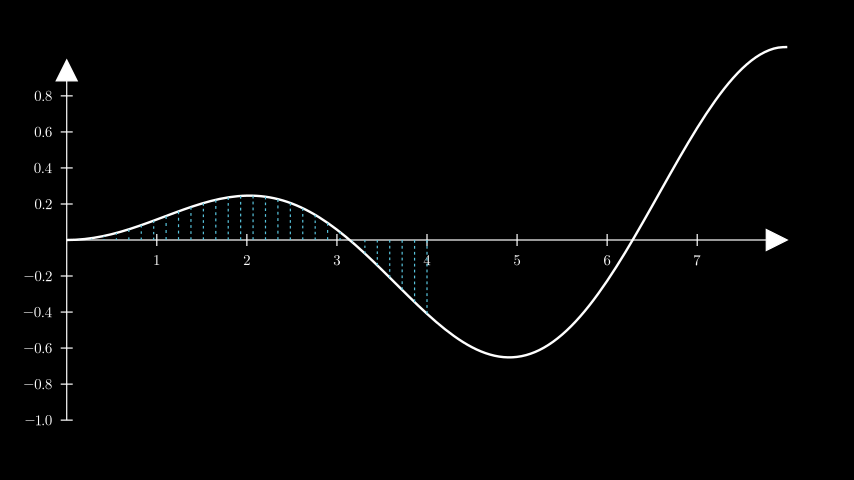
../_images/GetVerticalLinesToGraph-1.png

from manim import *

class GetVerticalLinesToGraph(Scene):
    def construct(self):
        ax = Axes(
            x_range=[0, 8.0, 1],
            y_range=[-1, 1, 0.2],
            axis_config={"font_size": 24},
        ).add_coordinates()

        curve = ax.plot(lambda x: np.sin(x) / np.e ** 2 * x)

        lines = ax.get_vertical_lines_to_graph(
            curve, x_range=[0, 4], num_lines=30, color=BLUE
        )

        self.add(ax, curve, lines)

get_x_axis_label(label, edge=array([1., 1., 0.]), direction=array([1., 1., 0.]), buff=0.1, **kwargs)[source]#

Generate an x-axis label.

Parameters

label (float | str | Mobject) – The label. Defaults to MathTex for str and float inputs.
edge (Sequence[float]) – The edge of the x-axis to which the label will be added, by default UR.
direction (Sequence[float]) – Allows for further positioning of the label from an edge, by default UR.
buff (float) – The distance of the label from the line.

Returns

The positioned label.

Return type

Mobject

Examples

Example: GetXAxisLabelExample ¶

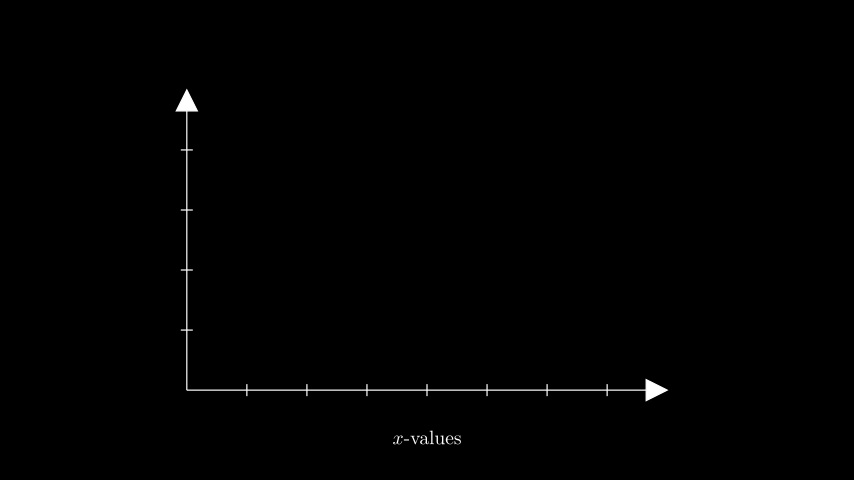
from manim import *

class GetXAxisLabelExample(Scene):
    def construct(self):
        ax = Axes(x_range=(0, 8), y_range=(0, 5), x_length=8, y_length=5)
        x_label = ax.get_x_axis_label(
            Tex("$x$-values").scale(0.65), edge=DOWN, direction=DOWN, buff=0.5
        )
        self.add(ax, x_label)

get_y_axis_label(label, edge=array([1., 1., 0.]), direction=array([1., 0.5, 0.]), buff=0.1, **kwargs)[source]#

Generate a y-axis label.

Parameters

label (float | str | Mobject) – The label. Defaults to MathTex for str and float inputs.
edge (Sequence[float]) – The edge of the x-axis to which the label will be added, by default UR.
direction (Sequence[float]) – Allows for further positioning of the label from an edge, by default UR
buff (float) – The distance of the label from the line.

Returns

The positioned label.

Return type

Mobject

Examples

Example: GetYAxisLabelExample ¶

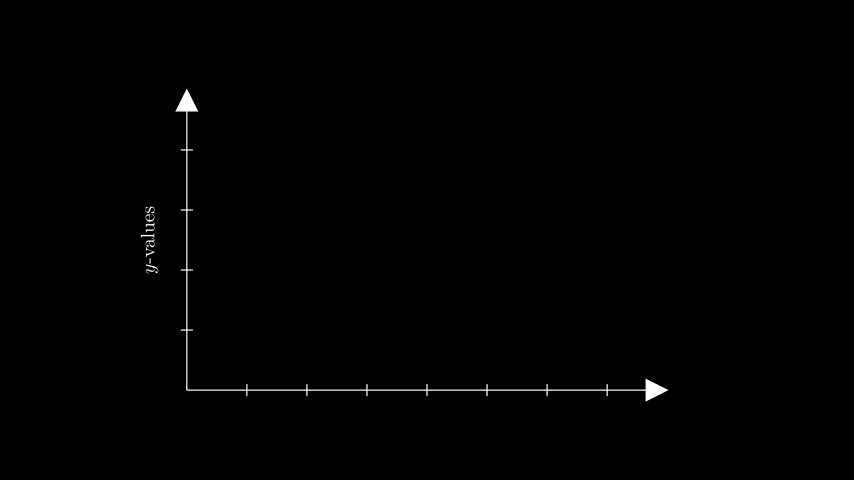
from manim import *

class GetYAxisLabelExample(Scene):
    def construct(self):
        ax = Axes(x_range=(0, 8), y_range=(0, 5), x_length=8, y_length=5)
        y_label = ax.get_y_axis_label(
            Tex("$y$-values").scale(0.65).rotate(90 * DEGREES),
            edge=LEFT,
            direction=LEFT,
            buff=0.3,
        )
        self.add(ax, y_label)

i2gc(x, graph)[source]#

Alias for input_to_graph_coords().

Parameters

x (float) –
graph (ParametricFunction) –

Return type

tuple

i2gp(x, graph)[source]#

Alias for input_to_graph_point().

Parameters

x (float) –
graph (ParametricFunction) –

Return type

ndarray

input_to_graph_coords(x, graph)[source]#

Returns a tuple of the axis relative coordinates of the point on the graph based on the x-value given.

Examples

>>> from manim import Axes
>>> ax = Axes()
>>> parabola = ax.plot(lambda x: x**2)
>>> ax.input_to_graph_coords(x=3, graph=parabola)
(3, 9)

Parameters

x (float) –
graph (ParametricFunction) –

Return type

tuple

input_to_graph_point(x, graph)[source]#

Returns the coordinates of the point on a graph corresponding to an x value.

Parameters

x (float) – The x-value of a point on the graph.
graph (ParametricFunction | VMobject) – The ParametricFunction on which the point lies.

Returns

The coordinates of the point on the graph corresponding to the x value.

Return type

np.ndarray

Raises

ValueError – When the target x is not in the range of the line graph.

Examples

Example: InputToGraphPointExample ¶

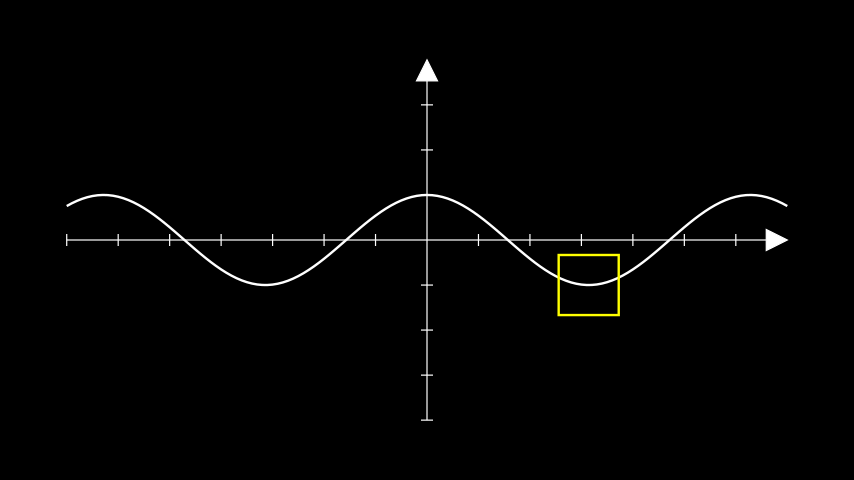
../_images/InputToGraphPointExample-1.png

from manim import *

class InputToGraphPointExample(Scene):
    def construct(self):
        ax = Axes()
        curve = ax.plot(lambda x : np.cos(x))

        # move a square to PI on the cosine curve.
        position = ax.input_to_graph_point(x=PI, graph=curve)
        sq = Square(side_length=1, color=YELLOW).move_to(position)

        self.add(ax, curve, sq)

p2c(point)[source]#: Abbreviation for point_to_coords()

plot(function, x_range=None, use_vectorized=False, **kwargs)[source]#

Generates a curve based on a function.

Parameters

function (Callable[[float], float]) – The function used to construct the ParametricFunction.
x_range (Sequence[float] | None) – The range of the curve along the axes. x_range = [x_min, x_max, x_step].
use_vectorized (bool) – Whether to pass in the generated t value array to the function. Only use this if your function supports it. Output should be a numpy array of shape [y_0, y_1, ...]
kwargs – Additional parameters to be passed to ParametricFunction.

Returns

The plotted curve.

Return type

ParametricFunction

Warning

This method may not produce accurate graphs since Manim currently relies on interpolation between evenly-spaced samples of the curve, instead of intelligent plotting. See the example below for some solutions to this problem.

Examples

Example: PlotExample ¶

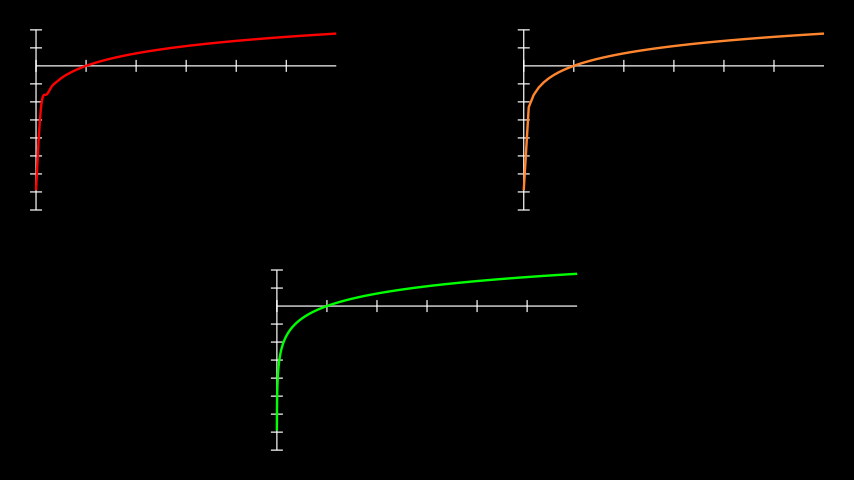
from manim import *

class PlotExample(Scene):
    def construct(self):
        # construct the axes
        ax_1 = Axes(
            x_range=[0.001, 6],
            y_range=[-8, 2],
            x_length=5,
            y_length=3,
            tips=False,
        )
        ax_2 = ax_1.copy()
        ax_3 = ax_1.copy()

        # position the axes
        ax_1.to_corner(UL)
        ax_2.to_corner(UR)
        ax_3.to_edge(DOWN)
        axes = VGroup(ax_1, ax_2, ax_3)

        # create the logarithmic curves
        def log_func(x):
            return np.log(x)

        # a curve without adjustments; poor interpolation.
        curve_1 = ax_1.plot(log_func, color=PURE_RED)

        # disabling interpolation makes the graph look choppy as not enough
        # inputs are available
        curve_2 = ax_2.plot(log_func, use_smoothing=False, color=ORANGE)

        # taking more inputs of the curve by specifying a step for the
        # x_range yields expected results, but increases rendering time.
        curve_3 = ax_3.plot(
            log_func, x_range=(0.001, 6, 0.001), color=PURE_GREEN
        )

        curves = VGroup(curve_1, curve_2, curve_3)

        self.add(axes, curves)

plot_antiderivative_graph(graph, y_intercept=0, samples=50, use_vectorized=False, **kwargs)[source]#

Plots an antiderivative graph.

Parameters

graph (ParametricFunction) – The graph for which the antiderivative will be found.
y_intercept (float) – The y-value at which the graph intercepts the y-axis.
samples (int) – The number of points to take the area under the graph.
use_vectorized (bool) – Whether to use the vectorized version of the antiderivative. This means to pass in the generated t value array to the function. Only use this if your function supports it. Output should be a numpy array of shape [y_0, y_1, ...]
kwargs – Any valid keyword argument of ParametricFunction.

Returns

The curve of the antiderivative.

Return type

ParametricFunction

Note

This graph is plotted from the values of area under the reference graph. The result might not be ideal if the reference graph contains uncalculatable areas from x=0.

Examples

Example: AntiderivativeExample ¶

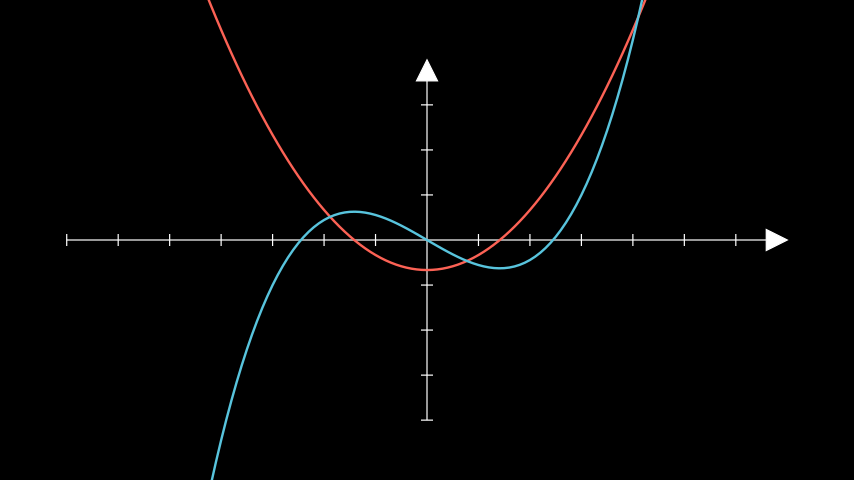
from manim import *

class AntiderivativeExample(Scene):
    def construct(self):
        ax = Axes()
        graph1 = ax.plot(
            lambda x: (x ** 2 - 2) / 3,
            color=RED,
        )
        graph2 = ax.plot_antiderivative_graph(graph1, color=BLUE)
        self.add(ax, graph1, graph2)

plot_derivative_graph(graph, color='#83C167', **kwargs)[source]#

Returns the curve of the derivative of the passed graph.

Parameters

graph (ParametricFunction) – The graph for which the derivative will be found.
color (Color) – The color of the derivative curve.
kwargs – Any valid keyword argument of ParametricFunction.

Returns

The curve of the derivative.

Return type

ParametricFunction

Examples

Example: DerivativeGraphExample ¶

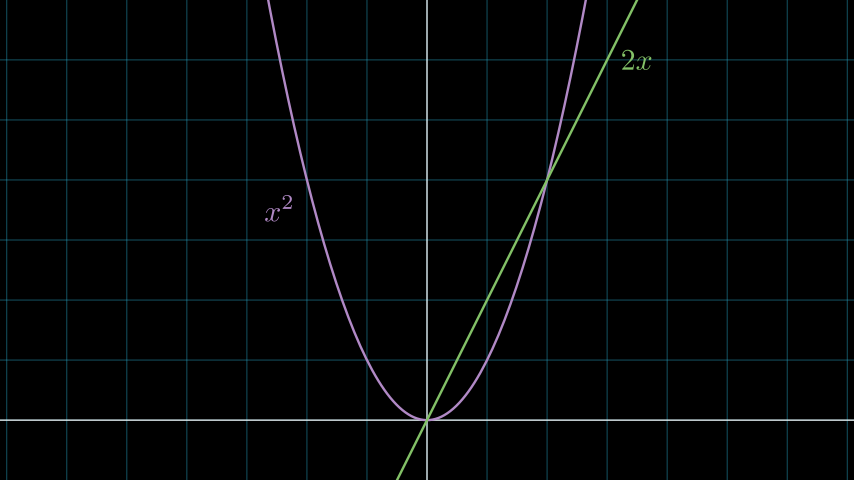
from manim import *

class DerivativeGraphExample(Scene):
    def construct(self):
        ax = NumberPlane(y_range=[-1, 7], background_line_style={"stroke_opacity": 0.4})

        curve_1 = ax.plot(lambda x: x ** 2, color=PURPLE_B)
        curve_2 = ax.plot_derivative_graph(curve_1)
        curves = VGroup(curve_1, curve_2)

        label_1 = ax.get_graph_label(curve_1, "x^2", x_val=-2, direction=DL)
        label_2 = ax.get_graph_label(curve_2, "2x", x_val=3, direction=RIGHT)
        labels = VGroup(label_1, label_2)

        self.add(ax, curves, labels)

plot_implicit_curve(func, min_depth=5, max_quads=1500, **kwargs)[source]#

Creates the curves of an implicit function.

Parameters

func (Callable) – The function to graph, in the form of f(x, y) = 0.
min_depth (int) – The minimum depth of the function to calculate.
max_quads (int) – The maximum number of quads to use.
kwargs – Additional parameters to pass into ImplicitFunction.

Return type

ImplicitFunction

Examples

Example: ImplicitExample ¶

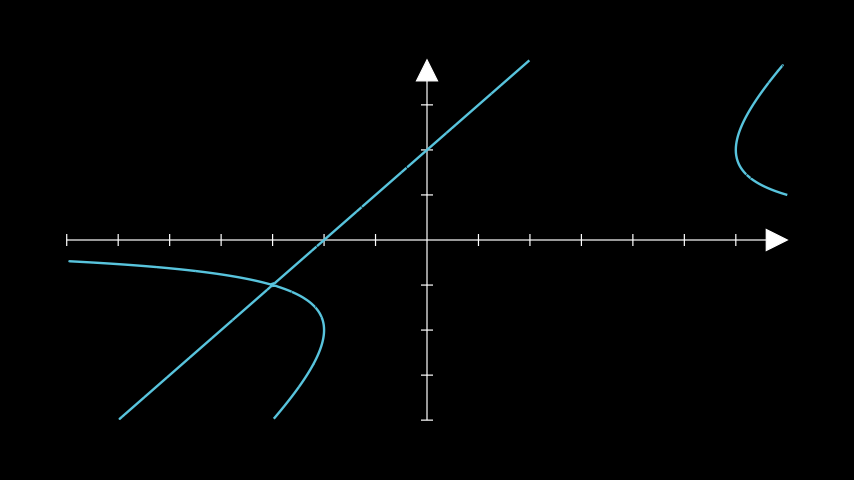
from manim import *

class ImplicitExample(Scene):
    def construct(self):
        ax = Axes()
        a = ax.plot_implicit_curve(
            lambda x, y: y * (x - y) ** 2 - 4 * x - 8, color=BLUE
        )
        self.add(ax, a)

plot_parametric_curve(function, use_vectorized=False, **kwargs)[source]#

A parametric curve.

Parameters

function (Callable[[float], ndarray]) – A parametric function mapping a number to a point in the coordinate system.
use_vectorized (bool) – Whether to pass in the generated t value array to the function. Only use this if your function supports it.
kwargs – Any further keyword arguments are passed to ParametricFunction.

Return type

ParametricFunction

Example

Example: ParametricCurveExample ¶

from manim import *

class ParametricCurveExample(Scene):
    def construct(self):
        ax = Axes()
        cardioid = ax.plot_parametric_curve(
            lambda t: np.array(
                [
                    np.exp(1) * np.cos(t) * (1 - np.cos(t)),
                    np.exp(1) * np.sin(t) * (1 - np.cos(t)),
                    0,
                ]
            ),
            t_range=[0, 2 * PI],
            color="#0FF1CE",
        )
        self.add(ax, cardioid)

plot_polar_graph(r_func, theta_range=[0, 6.283185307179586], **kwargs)[source]#

A polar graph.

Parameters

r_func (Callable[[float], float]) – The function r of theta.
theta_range (Sequence[float]) – The range of theta as theta_range = [theta_min, theta_max, theta_step].
kwargs – Additional parameters passed to ParametricFunction.

Return type

ParametricFunction

Examples

Example: PolarGraphExample ¶

from manim import *

class PolarGraphExample(Scene):
    def construct(self):
        plane = PolarPlane()
        r = lambda theta: 2 * np.sin(theta * 5)
        graph = plane.plot_polar_graph(r, [0, 2 * PI], color=ORANGE)
        self.add(plane, graph)

References: PolarPlane

plot_surface(function, u_range=None, v_range=None, colorscale=None, colorscale_axis=2, **kwargs)[source]#

Generates a surface based on a function.

Parameters

function (Callable[[float], float]) – The function used to construct the Surface.
u_range (Sequence[float] | None) – The range of the u variable: (u_min, u_max).
v_range (Sequence[float] | None) – The range of the v variable: (v_min, v_max).
colorscale (Sequence[[color], float] | None) – Colors of the surface. Passing a list of colors will color the surface by z-value. Passing a list of tuples in the form (color, pivot) allows user-defined pivots where the color transitions.
colorscale_axis (int) – Defines the axis on which the colorscale is applied (0 = x, 1 = y, 2 = z), default is z-axis (2).
kwargs – Additional parameters to be passed to Surface.

Returns

The plotted surface.

Return type

Surface

Examples

Example: PlotSurfaceExample ¶

from manim import *

class PlotSurfaceExample(ThreeDScene):
    def construct(self):
        resolution_fa = 16
        self.set_camera_orientation(phi=75 * DEGREES, theta=-60 * DEGREES)
        axes = ThreeDAxes(x_range=(-3, 3, 1), y_range=(-3, 3, 1), z_range=(-5, 5, 1))
        def param_trig(u, v):
            x = u
            y = v
            z = 2 * np.sin(x) + 2 * np.cos(y)
            return z
        trig_plane = axes.plot_surface(
            param_trig,
            resolution=(resolution_fa, resolution_fa),
            u_range = (-3, 3),
            v_range = (-3, 3),
            colorscale = [BLUE, GREEN, YELLOW, ORANGE, RED],
            )
        self.add(axes, trig_plane)

point_to_polar(point)[source]#

Gets polar coordinates from a point.

Parameters: point (np.ndarray) – The point.
Returns: The coordinate radius (\(r\)) and the coordinate azimuth (\(\theta\)).
Return type: Tuple[float, float]

polar_to_point(radius, azimuth)[source]#

Gets a point from polar coordinates.

Parameters

radius (float) – The coordinate radius (\(r\)).
azimuth (float) – The coordinate azimuth (\(\theta\)).

Returns

The point.

Return type

numpy.ndarray

Examples

Example: PolarToPointExample ¶

from manim import *

class PolarToPointExample(Scene):
    def construct(self):
        polarplane_pi = PolarPlane(azimuth_units="PI radians", size=6)
        polartopoint_vector = Vector(polarplane_pi.polar_to_point(3, PI/4))
        self.add(polarplane_pi)
        self.add(polartopoint_vector)

References: PolarPlane Vector

pr2pt(radius, azimuth)[source]#

Abbreviation for polar_to_point()

Parameters

radius (float) –
azimuth (float) –

Return type

ndarray

pt2pr(point)[source]#

Abbreviation for point_to_polar()

Parameters: point (np.ndarray) –
Return type: tuple[float, float]

slope_of_tangent(x, graph, **kwargs)[source]#

Returns the slope of the tangent to the plotted curve at a particular x-value.

Parameters

x (float) – The x-value at which the tangent must touch the curve.
graph (ParametricFunction) – The ParametricFunction for which to calculate the tangent.

Returns

The slope of the tangent with the x axis.

Return type

float

Examples

ax = Axes()
curve = ax.plot(lambda x: x**2)
ax.slope_of_tangent(x=-2, graph=curve)
# -3.5000000259052038